Talks
Featured Talk
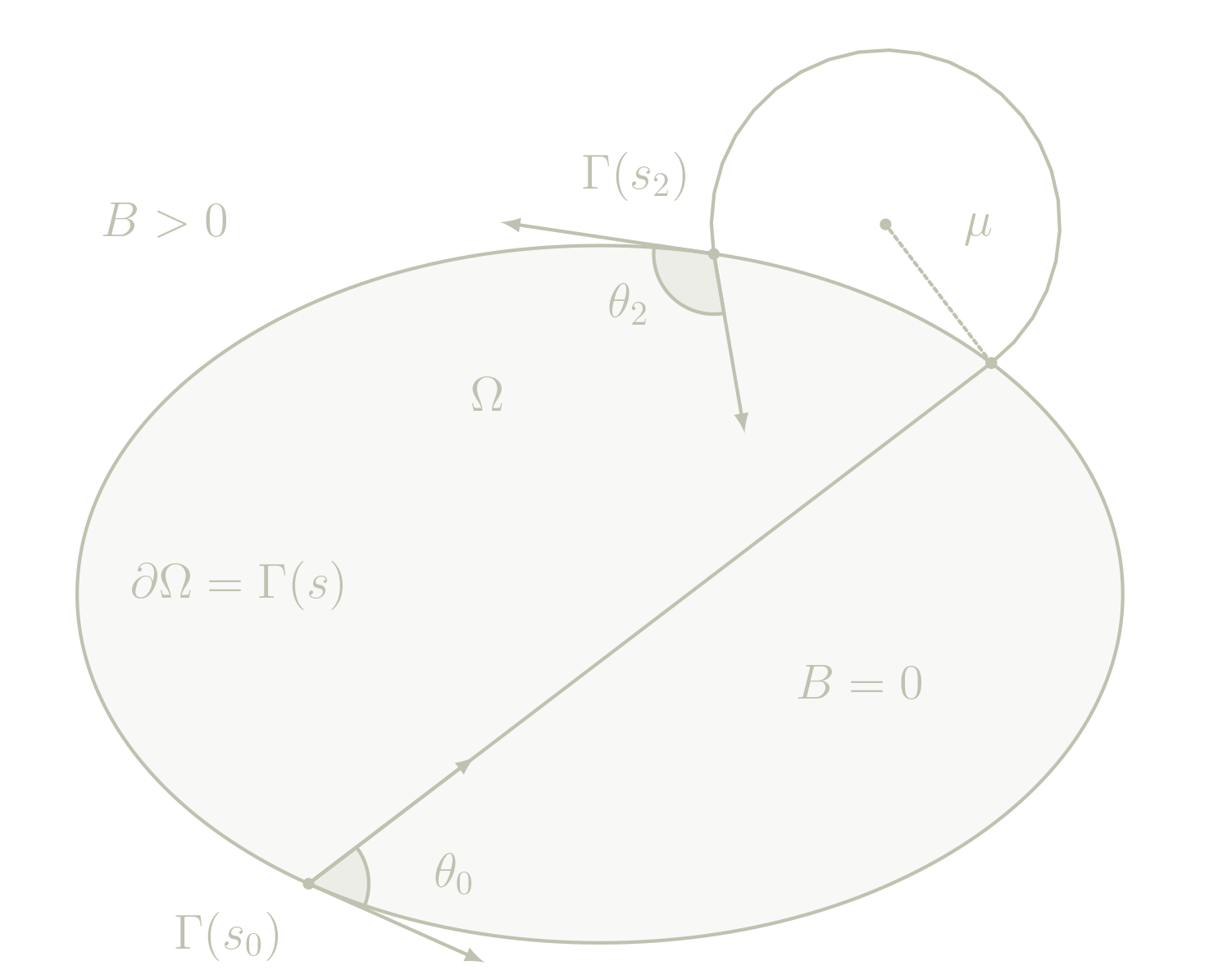
Minkowski Billiards on the Hyperboloid of One Sheet
Research Talks and Conference Presentations
67th Annual Meeting of the Australian Mathematical Society, University of Queensland, Brisbane, QLD, Aus, December 7, 2023: Dynamics and Periodicity Conditions for the Integrable Boltzmann System
11th Workshop on Integrable Systems, University of Sydney, Sydney, NSW, Aus, December 1, 2023: Dynamics and Periodicity Conditions for the Integrable Boltzmann System
Mathematical Billiards: at the Crossroads of Dynamics, Geometry, Analysis, and Mathematical Physics, Simons Center for Geometry and Physics, Stony Brook, NY, USA, October 30, 2023: Dynamics and Periodicity Conditions for the Integrable Boltzmann System
Cal Poly Simple Group, Cal Poly Math Department, San Luis Obispo, CA, USA, October 18, 2023: Mathematical Billiards, Part I
Symmetry for Group Actions in Differential Geometry, Matrix Institute, Creswick, Vic., Aus, May 25, 2022: Minkowski Billiards on the Hyperboloid of One Sheet
Nijenhuis Geometry and Integrable Systems, Matrix Institute, Creswick, Vic., Aus, February 14, 2022: A Tale of Two (Integrable?) Billiards
65th Annual Conference of the Australian Mathematical Society, University of Newcastle, Newcastle, NSW, Aus., December 9, 2021: Minkowski Billiards on the Hyperboloid of One Sheet
65th Annual Conference of the Australian Mathematical Society, University of Newcastle, Newcastle, NSW, Aus., December 8, 2021: Linear Stability of Periodic Trajectories in Inverse Magnetic Billiards
Differential Geometry, Billiards, and Geometric Optics (Online), CIRM Luminy, Fr., October 7, 2021: Inverse Magnetic Billiards: Periodic Orbits and Stability (poster)
SIAM Conference on Applications of Dynamical Systems (Online), Portland, OR USA, May 26, 2021: Linear Stability of Periodic Trajectories in Inverse Magnetic Billiards.
Mechanics Seminar, Mathematical Institute of the Serbian Academy of Sciences and Arts (Online), Belgrade, Ser., May 19, 2021: Minkowski Billiards on the Hyperboloid of One Sheet.
Sydney Dynamics Group Workshop, Jervis Bay, NSW, Aus., November 26, 2020: Open Problems in Magnetism and Billiards.
UNSW Applied Maths Seminar (Online), Sydney, NSW, Aus., July 9, 2020: Minkowski Billiards on the Hyperboloid of One Sheet
UNSW Pure Maths Seminar (Online), Sydney, NSW Aus., June 16, 2020: Playing Billiards with π
UC Santa Cruz Geometry and Analysis Seminar (Online), Santa Cruz, CA, May 29, 2020: Minkowski Billiards on the Hyperboloid of One Sheet
Sydney Dynamics Group (Online), Sydney, NSW Aus., April 24, 2020: Magnetic Variants of Billiard Systems
Joint Math Meetings AMS Contributed Paper Session on Dynamical Systems and Ergodic Theory, Denver, CO, January 17, 2020: Inverse Magnetic Billiards: A Survey
Joint Math Meetings AMS Special Session on Hamiltonian Systems, Denver, CO, January 15, 2020: Counting Billiard Collisions Using Angles Between Subspaces
63rd Annual Conference of the Australian Mathematical Society, Monash University, Melbourne, Aus., December 5, 2019: On the Dynamics of Inverse Magnetic Billiards
SIAM Conference on Applications of Dynamical Systems – DS19, Snowbird, UT, May 21, 2019: Billiards Inside, Circles Outside: Dynamics of a Charged Particle in a Piecewise-Constant Magnetic Field
AMS Western Sectional Meeting, Invited Speaker, AMS Special Session on Geometric Approaches to Mechanics and Control, Honolulu, HI, March 23, 2019: On the Dynamics of Inverse Magnetic Billiards
Joint Math Meetings, Baltimore, MD, January 16, 2019: Billiards Inside, Circles Outside: Dynamics of a Charged Particle in a Piecewise Constant Magnetic Field
AMS Fall Western Sectional Meeting, San Francisco, CA, October 27, 2018: Billiards Inside, Circles Outside: Charged Particles in a Piecewise Constant Magnetic Field
MAA Southern California-Nevada Fall Section Meeting, Cal Poly San Luis Obispo, November 15, 2015: Osculating Curves and the Tait-Kneser Theorem
College of Science and Mathematics Student Research Conference, San Luis Obispo, CA, May 25, 2011: Hereditary Matrices | View presentation slides
College of Science and Mathematics Student Research Conference, San Luis Obispo, CA, May 20, 2009: The Kakeya Needle Problem | View presentation slides
Outreach Talks
Monterey Bay Area Math Teachers’ Circle, Santa Cruz, CA, February 12, 2019: How Many Squares are in a Lattice?
Monterey Bay Area Math Teachers’ Circle, Santa Cruz, CA, April 10, 2018: A Four Numbers Game
Monterey Bay Area Math Teachers’ Circle, Santa Cruz, CA, October 10 & November 14, 2017: Finite Geometries — Infinite Fun! (Parts 1 and 2)
UC Santa Cruz Mathematics Department Undergraduate Colloquium, UC Santa Cruz, March 2, 2017: The Mathematics of Doodling
Monterey Bay Area Math Teachers’ Circle, Santa Cruz, CA, December 8, 2016: The Mathematics of Doodling
UC Santa Cruz Mathematics Department Undergraduate Colloquium, UC Santa Cruz, November 2 & 30, 2016: The ABC’s of the ABC Conjecture (Parts 1 and 2)
Monterey Bay Area Math Teachers’ Circle, Santa Cruz, CA, November 10, 2016: An Exploration of Area: Pick’s Theorem and More
Monterey Bay Area Mathematics Project Winter Math Conference: Leveraging the Power of Technology, UC Santa Cruz, February 27, 2016: GeoGebra in the Classroom
More talks
August 22, 2018
MSRI 5-Minute Talk
Two slides and five minutes. Seminars in this format are given by all participants at the MSRI semester program Hamiltonian systems, from topology to applications through analysis. This is a brief introduction to my research on inverse magnetic billiards.
April 24, 2020
Magnetic Variants of Billiard Systems, Sydney Dynamics Group
In this talk, we give a brief review of results and properties of mathematical billiards.
July 9, 2020
Minkowski Billiards on the Hyperboloid of One Sheet, UNSW Applied Maths Seminar
In this talk, we give a review of Euclidean and pseudo-Euclidean billiards in the plane and in d-dimensional space.