Research
My research is in geometry and dynamical systems, with a particular focus on billiards within confocal quadrics, magnetic variants of billiard systems, and integrable systems.
Research Publications
S. Gasiorek, Linear Stability of Periodic Trajectories in Inverse Magnetic Billiards. arXiv:2106.05676 [math.DS], 2023 (under review).
S. Gasiorek and M. Radnović, Periodic Trajectories and Topology of the Integrable Boltzmann System. Recent Progress in Special Functions, Contemporary Mathematics, vol. 807, Amer. Math. Soc., Providence, RI, pp. 111-130 (2024). DOI: 10.1090/conm/807.
V. Dragović, S. Gasiorek, and M. Radnović, Integrable Billiards on a Minkowski Hyperboloid: Extremal Polynomials and Topology. Sbornik: Mathematics, Vol 213, no. 9, pp. 34-69 (2022). DOI: 10.4213/sm9662.
V. Dragović, S. Gasiorek, and M. Radnović, Billiard Ordered Games and Books. Regular and Chaotic Dynamics, Vol 27, no. 2, pp. 132-150 (2022). DOI: 10.1134/S1560354722020022.
S. Gasiorek, On the Dynamics of Inverse Magnetic Billiards, Nonlinearity, vol 34, no. 3, pp. 1503-1524 (2021). DOI: 10.1088/1361-6544/abe2f1.
S. Gasiorek and M. Radnović, Pseudo-Euclidean Billiards within Confocal Curves on the Hyperboloid of One Sheet, Journal of Geometry and Physics. (2020) DOI: 10.1016/j.geomphys.2020.104032.
S. Gasiorek, Counting Collisions in an N-Billiard System Using Angles Between Collision Subspaces, Symmetry, Integrability and Geometry: Methods and Applications (SIGMA), Volume 16 (2020), No. 119, 13 pages. DOI: 10.3842/SIGMA.2020.119.
Articles, Books, and Other Content
S. Gasiorek, Integrability in Magnetic Variants of Billiard Systems, DSWeb The Magazine, SIAM, July 2020 Issue.
S. Gasiorek, On the Dynamics of Inverse Magnetic Billiards. https://escholarship.org/uc/item/4dn4d2z1, 2019 (Dissertation)
S. Gasiorek and D. Paquin, Number Theory, 6th Ed. (335 pages). Course text for Stanford Pre-Collegiate Summer Institutes course.
S. Gasiorek and D. Paquin, Logic & Problem Solving, 2nd Ed. (141 pages). Course text for Stanford Pre-Collegiate Summer Institutes course.
S. Gasiorek and T. Woolf, The Kakeya Needle Problem (Undergraduate Senior Thesis), 2009.
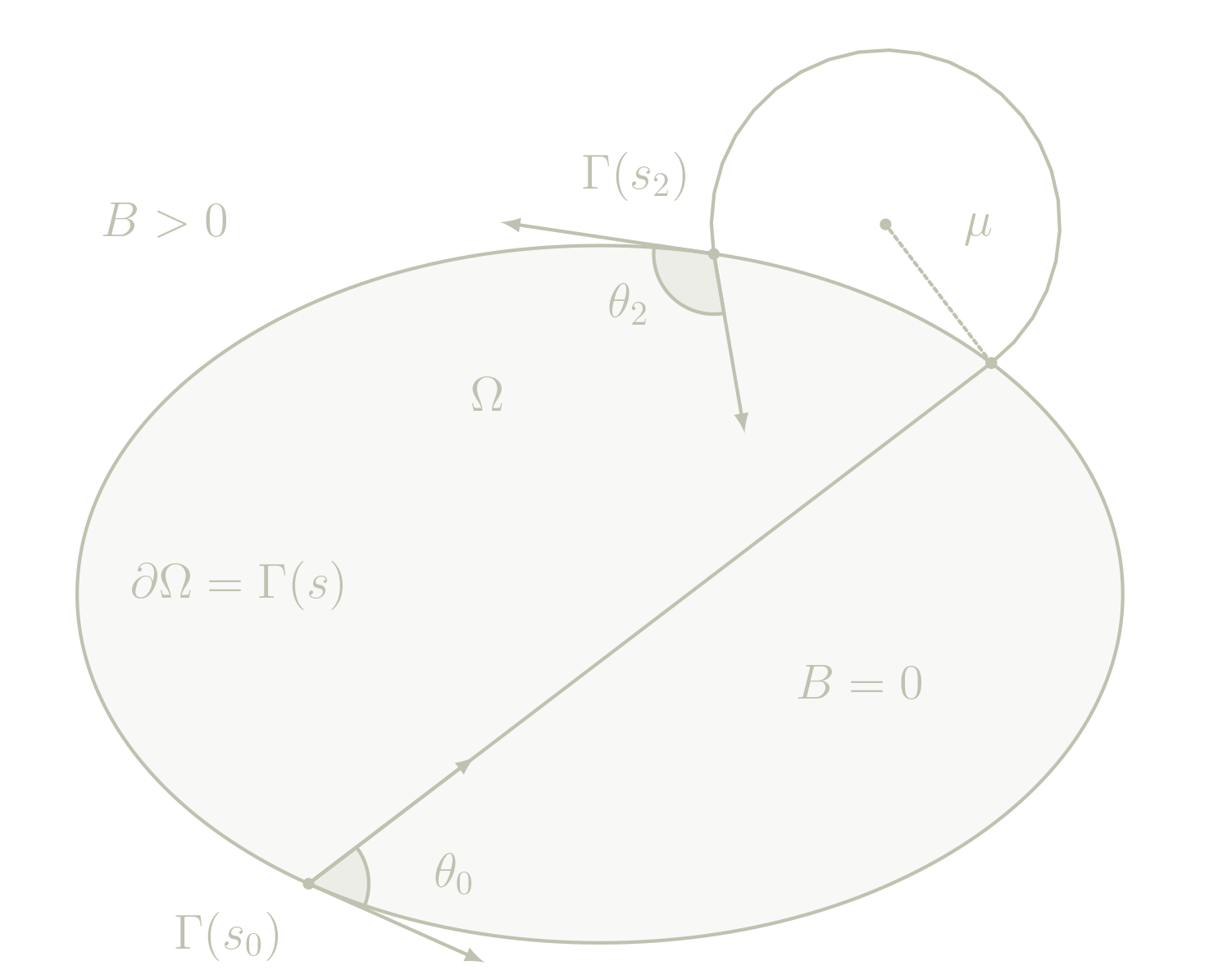
Inverse Magnetic Billiards
For my Ph.D. thesis, I studied a billiard problem that is a combination of standard Birkhoff billiards and magnetic billiards. If the billiard is seen as a charged particle under the influence of a magnetic field orthogonal to the plane that is 0 inside some convex domain Ω and B>0 outside Ω, then the motion of the billiard is a straight line inside Ω and circular arcs of fixed radius outside Ω. My research into this inverse magnetic billiard shows that some properties of standard Birkhoff billiards and magnetic billiards apply while others do not.
Billiards in Confocal Quadrics
Some of my recent work in billiards is joint work with Milena Radnovic (University of Sydney), and concerns the setting of billiards on surfaces and within confocal quadrics. For example, billiards on a hyperboloid of one sheet with the Minkowski metric move along geodesics until they reflect off of the curve of intersection of a confocal quadric and the hyperboloid, and this billiard flow can be shown to be integrable in the sense of Liouville. Further, we derive an algebro-geometric Cayley condition for the existence of periodic orbits and explore geometric consequences and connections to polynomial analogues to the periodicity conditions.
